Noah Rhee asks
Dear Professor,
A question professor, what is time dilation and how does it work? Furthermore, if time indeed is relative, how would this play into futuristic space colonization?
Thanks,
Noah Rhee
Oh, this is a doozy. Time dilation is something I’ve tried to avoid talking about because it’s one of those things that involves counterintuitive concepts that are particularly difficult to explain without using reams and reams of awful, awful maths. However this is by far the most polite question I’ve been asked so far, and so I shall make a special effort just for you.
There are two types of time dilation: time dilation which arises from differences in relative velocity (i.e. going really really fast compared to a “stationary” observer), and gravitational time dilation caused by particularly heavy celestial bodies such as planets and stars. The gravitational type is pretty much impossible for me to explain since it arises as a consequence of general relativity, and I don’t understand that anywhere near enough to feel comfortable describing it. Happily the type you appear to be asking me about is time dilation due to a difference in relative velocity, which I do understand fairly well and which furthermore has several simple prepackaged metaphors and thought experiments to aid in its explanation.
This particular type of time dilation is a result of the invariance of the speed of light, and the usual analogy used to get this across is the hilariously quaint boy with a peashooter riding a bike. The boy is riding his bike at five metres per second. To a stationary observer moving at zero metres per second, the boy appears to be riding his bike at five metres per second. Sounds straightforward, right? The boy then fires a pea out of his peashooter which is also travelling at 5 ms-1. To the stationary observer this pea was already going at 5 ms-1 before it was fired since the boy was carrying it along with him. After being fired it has a combined velocity of 5 ms-1 + 5 ms-1 = 10 ms-1 – with respect to the stationary observer’s frame of reference. With respect to the boy’s frame of reference, the pea is moving away from him at the 5 ms-1 at which he fired it, and so as far as he’s concerned the pea has a velocity of 5 ms-1.
This analogy explains how frames of reference work and how different observers can see the same object moving at different relative velocities. As far as light is concerned, though, it travels at exactly the same velocity relative to all observers and in all frames of reference. Light travels at 300,000 km s-1, or c. We can explain the invariance of the speed of light using our above analogy, except this time we replace the boy’s peashooter with a flashlight and accelerate him up to a relativistic velocity of, I don’t know, 0.1c (10% of the speed of light). To the stationary observer the boy is travelling at 0.1c. The boy turns on his flashlight. He sees the light travelling away from him at c, the speed of light, just as he saw the pea travelling away from him at 5 ms-1. But the stationary observer doesn’t see the light travelling at the combined velocity of the boy and the light; that is, he doesn’t see it travelling at a velocity of c + 0.1c = 1.1c. He sees the light travelling at c, just like the boy. This is what we mean when we say the speed of light is invariant; everyone sees it travelling at c no matter where they are or how fast they are moving.
In order for the universe to accommodate this invariance something else somewhere has to make some concessions so that the fabric of the universe doesn’t fall askew like a fat man’s bath towel, and that something is time. Time dilation is the universe’s way for compensating for the invariance of the speed of light; light can be the same speed for everyone just so long as they perceive the passage of time at different rates depending on where they are and how fast they are going. If you want a why as to the invariance of the speed of light I’m afraid I can’t help you. It’s one of those deep cosmological things that has been proven experimentally (most notably by the Michelson Morley interferometer experiment) but for which we don’t quite know the explanation. However, now that we know that it is invariant we can figure out the whys and hows of time dilation using another simple thought experiment: the light clock. I’m cribbing this particular example from Wikipedia but it’s one which is used to teach first-year undergraduate students the world over, so it’s not like they’ve got copyright on the method.
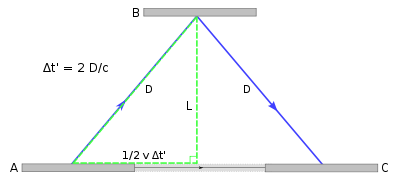
Imagine a beam of light bouncing between a pair of mirrors. Each time the light bounces from one mirror to the other and back again, the clock “ticks” once. The time period between ticks will be the time it takes for the light to do this, i.e. twice the distance L separating the two mirrors (since the light has to cover this distance twice) divided by the speed of light, C.
That is the length of the tick when both mirrors are stationary with respect to the observer. When two things are stationary with respect to one another they have the same reference frame, and there is no time dilation apparent between the two. In order to observe the effects of time dilation we’re going to have to set one mirror in motion.
In this setup the bottom mirror is moving from left to right with a velocity v. The top mirror remains stationary. The path of the light is now angled in comparison to the light from the first clock, meaning the light has to travel a longer distance D. The time it takes for this clock to “tick” once will therefore be
If we know the velocity of the bottom mirror v we can use simple trigonometry to work out the value of td in terms of L, v and c – i.e., in terms comparable to the tick of the stationary clock.
The perpendicular distance separating the mirrors is L. The bottom mirror is moving at velocity v. The bottom mirror is moving from left to right at the same rate as the beam of light moves from left to right (it has to in order to be there to receive the light at the end of the “tick”), so when the beam of light strikes the top mirror the bottom mirror will be directly underneath it. Exactly half the tick time period td will have elapsed once this happens, so the total distance the bottom mirror will have moved from left to right at this point will be its velocity multiplied by the time it has spent travelling at this velocity, or
These three vectors form a right-angled triangle, with D as the hypotenuse. Pythagoras’s theorem states that the square of the length of the hypotenuse will be the sum of the square of the lengths of the other two sides of the triangle, or
Taking the square root gives
And we can then substitute this value for D back into our original equation for td above (td = 2D/c).
We then do some extremely convoluted rearranging and cancelling out to get an equation of the form
But wait, didn’t we say earlier that 2L/c was equal to the tick period of the first stationary clock t?
And that is how you get your time dilation of a moving body as it appears to a “stationary” observer. Not seeing it? Well, hopefully I can help you out there.
First, why does this happen? It’s to do with the invariance of c and the different reference frames mentioned above. If we lived in a world where time was invariant (i.e. td = t without any of that other crap) then the speed of light would not be absolute, and we’d have a similar situation to the one with the boy and his peashooter. To a stationary observer the light in the stationary clock would travel at c, while the light in the moving clock would travel at c plus some component of the left-to-right velocity v. However, it does not do this. The light in the moving clock moves at c as well. In order for the light in both the stationary and moving clocks to move at c even though you have this additional velocity vector v added in to the moving clock, you have to slow down time in the moving reference frame. It’s not quite a correct way of thinking about it, but you could say that light moving with the “faster” velocity c + v at this slower rate of time appears, to an outside observer, to be moving at the normal speed of light c. The time dilation cancels out any added velocity vectors from the perspective of a stationary reference frame and ensures light always moves at c. It’s a physical necessity even though it seems ridiculously counterintuitive to our sluggish human perception of time.
What the above equation is saying is the tick period of a moving clock td will be equivalent to the tick period of the stationary clock t divided by the term in the square root. C is a really, really big number (3 × 108 ms-1) so if the velocity of the moving clock v is the sort of thing we’re likely to see in our everyday lives (velocities on the order of 10-1000 ms-1) then v2/c2 is going to be really goddamn small — it’s practically nothing, and when you subtract nothing from something you’re still left with the original something. This means 1 – v2/c2 is going to be effectively 1, the square root of 1 is 1, and so the equation shakes out to td = t. In other words, for relatively small velocities (small in comparison to c) time dilation will be almost non-existent. This is why we don’t notice time dilation as we walk around on the surface of the Earth, and even satellites orbiting at several kilometres per second need extremely sensitive clocks to measure the effect of time dilation (although it is relevant). It is only when you get up to velocities approaching a significant fraction of the speed of the light that the v2/c2 term in that equation is anywhere near big enough to put a significant dent in the 1 when subtracted from it.
Once you’re there, though, things start getting interesting. The 1 – v2/c2 term in the square root becomes appreciably smaller than 1. When you divide t by a number smaller than 1, it has exactly the same effect as multiplying it by a number larger than 1. In other words, for every tick td the dilated clock makes, a stationary clock will make 1.1 or 1.25 or 2 ticks. And if a stationary clock is ticking twice for every tick of a dilated clock, then if you’re in the same reference frame as the dilated clock time will effectively be moving forward at half the rate for you as it would for somebody in a stationary reference frame. The stationary person will age at twice the rate you do and live their life at twice the pace. That’s time dilation. I was going to make my own graph of this using FABULOUS EXCEL TECHNOLOGY but it turns out Excel sucks, and so I resorted to stealing this one off the usual source.
The X-axis shows the speed of an object expressed as a fraction of the speed of light c (i.e. 0.1 is equivalent to 0.1c). The Y-axis shows td/t (or how many “ticks” a stationary clock will make in the time it takes for a dilated clock moving at the relevant speed to make one tick) as the speed increases. The graph shows that even somebody moving at 0.5c – half the speed of light — will experience very little time dilation. It’s only when you get to 0.6c that it becomes noticeable, and 0.9c that it becomes significant. However, once you’re past 0.9c the amount of time dilation you experience begins to increase very very quickly, trending to infinity as you approach the speed of light.
So that’s time dilation. It is a thing that happens, and that we know happens. We have measured it many times. We have to take time dilation into account when plotting the long-term trajectories of interplanetary probes, even though the amount of time dilation the probes experience is tiny. I think the GPS satellites even have to compensate for time dilation when doing their routine station-keeping maneuvers. But what does it mean for space colonisation?
At the moment, very little. We do not need to move at relativistic velocities to get around the solar system – indeed, the drastic acceleration required to get to relativistic velocities in the timescale required would crush any human crew on board a relativistic spacecraft to paste. Time dilation, if it is a factor at all, will only become a factor if and when we get around to sending out colonisation parties to nearby stars – and this is a journey that will take so long that some form of suspended animation will be necessary no matter how we do it. It would take decades (or longer) just to accelerate to a velocity where time dilation becomes relevant (and this is leaving aside the fact that you have to slow down again before you can stop at your destination), so while we’re likely to be travelling interstellar distances at relativistic velocities, time dilation is not a get out of jail free card for people on board to age so slowly they can weather the trip in a human lifetime. It will be a factor, but it will be a factor in the same way it is for the GPS satellites: a technical hurdle that must be taken into account rather than a neat form of time travel. After all, if your trip is taking longer than a human lifetime it doesn’t much matter for the person on board whether it takes 300 years or 30,000; they’re still going to be dead at the end of it without some kind of space-magic stasis technology.
Wow, this really reminded me why I don’t do sums on this blog. Anyway, I hope this at least made time dilation a little clearer for those of you who have stuck with me through to the end. Thanks for reading!






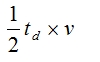


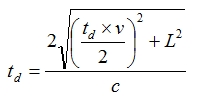

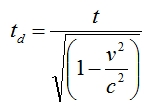


PS – Joe, if you’re reading this, I do have your question on LFTR reactors and it is a good question. However, I’d like to tie it into a series of posts I’m planning to write about nuclear reactors in general and so it’s going to take a week or two for me to get around to it.
I’ve been reading regularly since I found a blog about a fellow scientist/engineer addicted to 4x sci-fi games. Anyways, thanks for the update. Looking forward to the post series!
Yeah, I very much enjoyed this. In spite of the sums and equations. It is very nice to get a bit of a clearer view of how the mundane time dilation stuff works.
I don’t like littering posts with sums and equations, but in this case you need to see how time dilation pops out of the maths if you assume c is invariant to understand it.